

Once you have found that Constant, A, and B that maximizes that sum, you can then plug the Constant, A, and B into the Logit equation:Īfter this, you have the correct Logit (L), which can then be plugged into the probability equation: P(X) = e**L/ (1+e**L) and you have the most accurate probability of whether your new prospect will purchase. When you have found the ideal combination of (Constant, A, and B) that makes P(X) its most accurate for as many previous prospects as possible, the sum of all **(1-Y) ] equations will be maximized. The attached video shows this being performed. In fact you can consider Excel to be your logistic regression software. It is best to use a tool like the Excel Solver. Ultimately what we are doing is determining the Constant, A, and B that will maximize the sum of all P(X)**Y * **(1-Y) equations that we have calculated for each previous prospect. The equation P(X)**Y * **(1-Y) is maximized when P(X) approaches 1 (100%) when Y=1 and when P(X) approaches 0 when Y = 0. P(X) is the probability equation and P(X) = e**L/ (1+e**L) Y = 1 if the prospect purchased and Y = 0 if the prospect did not purchase. For each of our previous prospect, we construct the following equation: We have recorded the age, gender, and whether each prospect purchased for all of our hundreds of previous prospects. We go back to our original customer and prospect data. So, the question is: How do we solve for Constant, A, and B? L can then be plugged into the probability equation P(X) above and we have the probability of the prospect purchasing. Once we have solved for these, we have solved for L. L is the Logit and L = Constant + A*Age + B*Gender As a logistic regression example, if we were trying to predict the probability of a new prospect buying based upon the prospect's age and gender, then the equation for the Logit (L) would be the following:
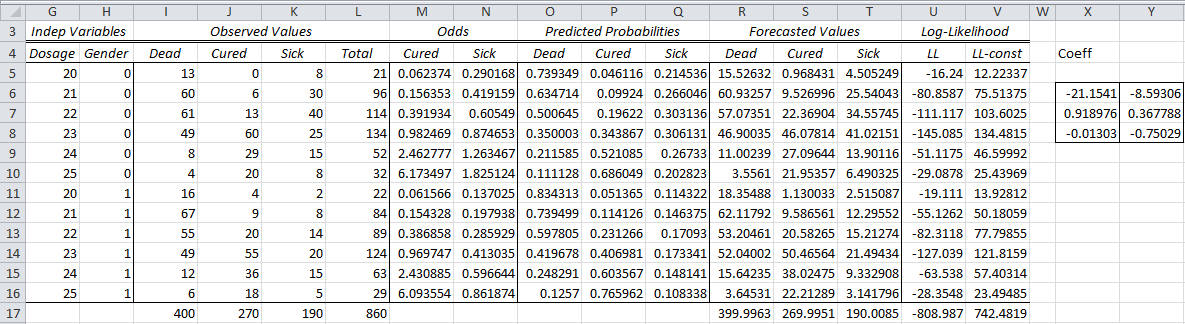
The formula for L depends on the input variables. The only variable in the above equation is L. The probability of the event occurring is given as follows: Here is how the calculation is done (without wasting much time on theory): Marketers often use logistic regression to calculate the probability of whether or not a prospect will purchase. For example, one medical use of logistic regression might be used to predict whether a person will have a stroke based upon the person's height, weight, and age. Logistic regression is used in social and medical sciences. Logistic regression is often called logit regression, the logistic model, or logit regression. The input variables (the predictor variables) can be numerical or categorical (dummy input variables). Logistic regression analysis is performed by fitting data to a logit regression function logistic curve.
Logistic regression is normally used to calculate the probability of an event occurring. Simple Explanation of How Logistic Regression Analysis Is Performed
#LOGIT REGRESSION EXCEL HOW TO#
Video - How To Do Logistic Regression in Excel Nankai-Grossman Center for Health Economics and Medical Insurance
#LOGIT REGRESSION EXCEL MANUAL#
I will use your manual as a reference for my MBA course this summer." If you're a student of business statistics, this e-manual is worth WAY more it's priced. I'm really glad that I came across the manual. It has really made my work with statistics a LOT easier. The explanations and videos in the manual are excellent. "I really like the Excel Statistical Master. Unlike the indecipherable jargon in the countless books I have wasted money on, the language in this book is plain and easy to understand. "After years of searching for a simplified statistics book, I found the Excel Statistical Master. Easy to follow and written so even a child could understand some of the most complex statistical theories. Little did I know, this book has everything I could ever want to know about business statistics. "We just started building statistical excel spreadsheets for our direct mail and online marketing campaigns, I purchased Excel Statistical Master to help fill in some of the blanks.


 0 kommentar(er)
0 kommentar(er)
